Brooks, Smith, Stone, Tutte (Part I)
The Trinity Four
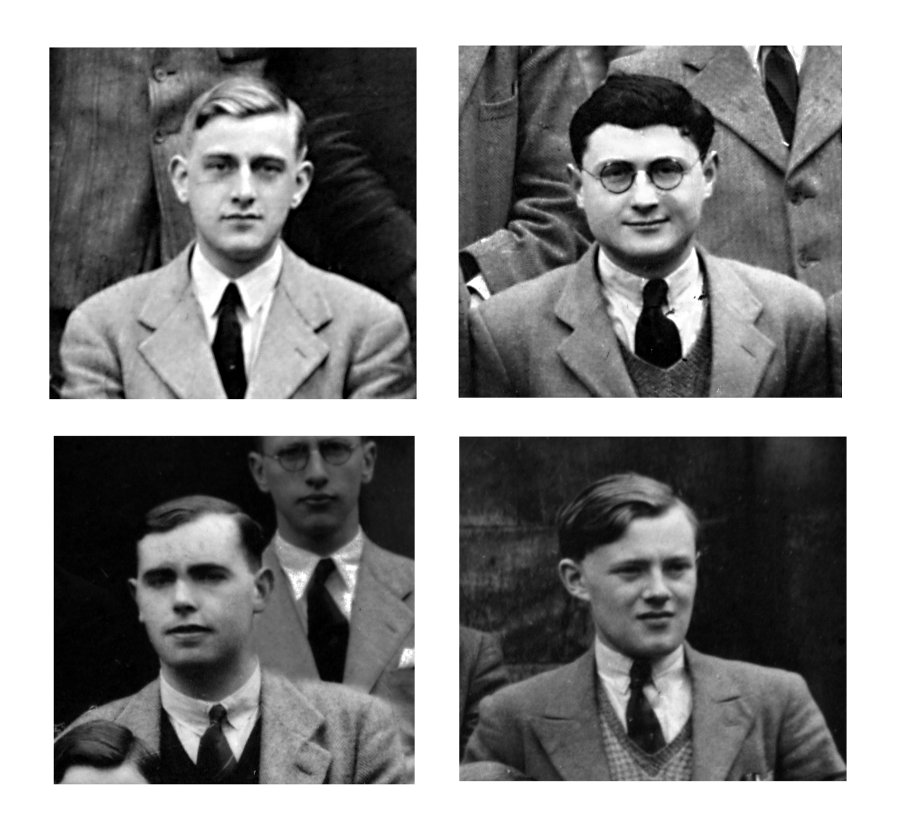
The Trinity Four; Brooks, Smith, Stone & Tutte (Cambridge 1938); photos supplied by Iain Strachan (private collection 2013)
Brooks, Smith, Stone and Tutte were all consulted and contributed material and recollections to Jasper Skinner's book 'Squared Squares, Who's Who & What's What' (i). Thanks to Jasper Skinner for allowing me to quote parts of his book here. Jasper's book has an anecdote about how Smith met Brooks on their first day at Trinity. At the Combinatorial Conference at Waterloo, held to celebrate the sixtieth birthday of W. T. Tutte, Smith describes the same meeting and how Brooks, Smith, Stone and Tutte came to collaborate;
"An essential first step took place in 1935, when 2 first-year mathematics students happened to be walking down Broadhurst Gardens, in northwest London. They met, recognised each other by sight, and indulged in a highly intellectual conversation:
Student 1: What are you doing here?
Student 2: I live here (long pause). What are you doing here?
Student 1: I live here (even longer pause)? I have some shopping to do. Goodbye.
Student 2: Goodbye
The following term they met at Trinity College, Cambridge, and found that their respective names were Arthur Stone and Cedric Smith . Now, because of a misprint in the timetable, Cedric Smith had found himself, together with another freshman named R. Leonard Brooks, attending an advanced lecture (on almost periodic functions) on his very first day of lectures; and they became friends:"
The version communicated later to Skinner on this meeting was more colourful;
"Just over 55 years ago I found myself, with great anticipation and excitement, attending my first ever lecture. It was on geometry and the lecturer talked about adding points together and multiplying them by numbers. That was not geometry as I understood it. At the end of the lecture I said to the young man next to me, 'That was confusing.' (The young man of course was Leonard Brooks a private man with a hearty, robust laugh.) "He replied, 'I thought it was a very good lecture. When is the next one?'. There was some momentary confusion and they entered lecture room 1 expecting a lecture from Mr Besicovitch on real variables. CABS recalled that they had just settled down when in came the lecturer and began with the following, "IVANKEHIOSUTOKL STMNDEJLSZIRTUNG..." CABS gasped, thinking to himself, "It must be English, surely?". It took him fifteen minutes to recognise even one word. Half an hour into the lecture CABS and RLB simultaneously concluded they were in the wrong class. They were! It was a post-graduate lecture on "almost periodic functions". The two retired to the young man's dormitary where he introduced himself as Leonard Brooks."(i)
CABS continues the story on how how all four met up; "So Cedric Smith introduced Leonard Brooks to Arthur Stone. And to reciprocate, Leonard Brooks introduced us all to a chess-playing friend, a chemist named William Tutte."
Rowland Leonard Brooks (RLB) (1916 - 1993)

Leonard Brooks 1938
Rowland Leonard Brooks, known as Leonard Brooks, was born February 6, 1916, in Lincolnshire, England. After meeting Smith, Stone and Tutte at Trinity College Cambridge he pursued the problem of squaring the square with them. At Trinity he proved an important theorem in graph theory, now known as Brooks' Theorem [ii],[2].
Later he became an Income Tax Inspector in England [VII]. He continued his interest in squared squares throughout his life. He held the low order simple squared square record for several years. He also was the first to find a 1x2 simple perfect squared rectangle - a 'squared domino' [3], at the time this problem was second only in importance to the problem of finding the lowest order simple perfect squared square.
Brooks was a very private man and did not wish for biographical information to be made available. He died in 1993. Brooks' Theorem is still a key result in the theory of graph colouring, and Brooks' role in investigating squared squares with Smith, Stone and Tutte is well known and documented. Rowland Leonard Brooks died on 18 June 1993.
Cedric Austin Bardell Smith (1917-2002)

C.A.B. Smith (CABS)
In 1935 Cedric Austin Bardell Smith (CABS) set foot on the campus at Trinity College Cambridge. He had failed a vital examination but was generously accepted. CABS was born in Leicester, England (about three minutes walk from the home of the eminent author C. P. Snow) on February 5, 1917. He remembers his parents as broad minded, and though religious (his father a Spiritualist and mother a Congregationalist) they did not impose their views on their children. His father (who he described as happy to the end of his days) was an engineer with his own business but suffered financial reverses following the 1929 market crash and ensuing depression.
CABS was educated at Wyggeston School until 1929 when the family moved to Tooting in southwest london. His education continued there at Bec School for three years. While at Bec, an enlightened relgious teacher made him aware of various religions and sects. It was at this time CABS developed his inital interest in the Quaker religion. His family moved next to West Hampstead, in northwest London where he attended University College School for three years.
Upon admission to Trinity, with a small grant, his scholastic achievements netted him a college scholarship in 1937 which allowed to finish work towards his degree. The same year he adopted the Quaker religion which he continues to this day. In 1938, he graduated first class and was awarded a Research Scholarship and for three years did research on statistical problems culminating his studies with the degree of Doctor of Philosophy and a "Rouse Ball Studentship" for three years of post-doctoral study.
He was unable to pursue post-doctoral study because of the outbreak of the war, but initially he trained a relief worker and then worked three years as a hospital porter. After the war he spent a few months packing parcels of clothing being dispatched to devasted areas.
CABS then resumed his academic career applying for a post at Galton Laboratory (Genetics), University College London and in 1946 became Assistant Lecturer, in 1948 Lecturer, 1957 Reader and in 1964 Professor, officially retiring in 1982. In 1985 he noted the retirement has had no noticeable effect on the demands on his time. "Cedric Smith fondly imagines that he is at present Emeritus Professor of Biometry, UCL, Chairman of the Conflict Research Society, Joint Editor of Colson News (Two-way Numbers), Joint Editor of Annals of Human Genetics, son-in-law of a Hungarian expert on Summability, and an Out-Patient in University College Hospital. He thinks that he once was TMS Secretary, porter at Addenbrookes Hospital, Chairman of the British region of the Biometric Society, Secretary of the Research Section of the Royal Statistical Society, Treasurer of the Friends Peace and International Relations Committee, and a Youth Hosteller. He also thinks he is interested in Graph Theory, Theory of Games, Phonetics, Subjective Probability, Elliptic Functions, Iteration, Finger Prints, Orthology and Matroids. However, all that can only be self-delusion, because an authoritative paper by Descartes in the Journal of Recreational Mathematics demonstrated conclusively that C.A.B. Smith is only a CAmBridge myth, being no more than one of many pseudonyms adopted by the prolific Italian mathematician, Prof. Carla Rossi of the University of Rome."[2]
He first met his wife Piroska while attending St. Andrews Mathematical Colloquium, attended by his friend, Hungarian mathematician Dr. Paul Vermes, (born 31 July 1897, died 26 February 1968). Fortunately, Dr. Vermes' daughter, Piroska (born 18 June 1921), was also in attendance. CABS found her beautiful and charming. Four years later the three attended the next Colloquium and two years later, she and Cedric married and exchanged their personal wedding bands for men and women to each other.. They had one son, Lionel Paul Adam Smith, he was born at University College Hospital in London on 5th March 1961. Piroska Smith passed away 9 November 2000. Cedric Austin Bardell Smith died 10 January 2002,
View from the lab: a mastermind for a number of reasons Obituary article by Steve Jones, Professor of Genetics at University College London.
"This afternoon I plan to attend the funeral of my old friend and colleague Cedric Smith, who has died at the age of 84.
Cedric was a member of that disappearing breed of academics who could immerse themselves in work, rather than in filling up forms. Like many mathematicians, he was fond of jokes. Thus: what did Jesus mean when he said, "Heaven equals ax2 + bx + c"? I, as an innumerate biologist, had to be let into the secret: it's a parabola.
Cedric Smith invented a new system of arithmetic (which worked well with a bit of practice) based on counting from one to five, and replacing all numbers greater than that with the same number subtracted from 10 and printed upside-down. He also developed the standard statistical methods now used to map genes on to chromosomes and wrote a solid text book called Biomathematics. His most celebrated works, though, are often not attributed to him, for they appeared under the pseudonym of Blanche Descartes, a mythical Frenchwoman still often referred to in the mathematical literature.
Descartes was in fact a committee consisting of a non-empty set (as mathematicians would say) drawn from the four members, Cedric included, of the Trinity College Mathematical Society, who met when they went up to Cambridge in the 1930s. All became mathematicians, and one, Bill Tutte (who is still with us), was an important part of the Bletchley Park code-breaking team.
Blanche (or her avatars) published on a great variety of subjects. One afternoon, the group were fiddling with the problem of whether it was possible to cut a square into a number of smaller squares, with no two of them the same. After a lot of scribbling, it turned out that it was, and the squared square had been invented. They seem pretty abstruse, but are useful in the design of electrical networks.
The smallest possible one - not discovered until 1978 - consists of 21 separate squares, all different, which can be assembled into the larger unit. The group also came up with "Blanche's Dissection", the simplest way to divide a square into rectangles of the same area but different shapes. Now, dozens have been found and such tiling problems, as they are called, are part of modern mathematics.
Another of their interests involved what became known as the Counterfeit Coin Problem: you are given a shilling's worth of pennies (that's 12, for our younger readers), one of which is counterfeit and differs from the others only in that it is slightly too heavy. Using a pair of balance scales, what is the smallest number of weighings that will pick out the false coin? The answer is 3; and Blanche came up with the solution that works for any number of coins.
That too seems a little obscure, but was one of the first developments in what became known as search theory, a branch of mathematics now much used in computing, economics and in looking for crashed aircraft and lost mountaineers.
That pleased Cedric, for he was a Quaker and passionate pacifist, who was much involved in the peace studies movement and in the mathematical analysis of wars, and what might be done to stop them. He would have been less happy about its other applications, which are heavily military and were used in the recent attacks upon Afghanistan.
In the final paragraphs of Biomathematics he wrote what can now be read as his epitaph; that "Mathematics needs to be wisely guided. Like other instruments, it can be used properly and with discrimination, or foolishly and inappropriately. Even the finest and most effective instrument can be put to base as well as to noble purposes, to impoverishment and destruction as well as to the enlargement of life and the creation of beauty."
Blanche Descartes has continued to publish papers (albeit under the identities of her individual members) to this day; and, in a forthcoming issue of the Mathematical Gazette, Cedric is poised to reveal - alas, posthumously - the secret of how she got her name."[d]
Obituary Cedric Smith (1917-2002) by Newton Morton
"Born during the Great War, Cedric Austen Bardell Smith was committed to Quaker pacifism, mathematics, and statistical genetics. As a Cambridge undergraduate he was one of the four members of the Trinity Mathematical Society which attacked arcane questions. They solved the problem called "Squaring the Square", demonstrating that a square is composed of smaller squares, all of different sizes, and applied this to electrical networks with varying resistance. He wrote amusing stories, poems and songs, usually about mathematics or mathematicians, under the pen-name of Blanche Descartes.
An attempt much later by a graduate student to contact the distinguished lady herself uncovered two facts: the inquiry received a prompt reply in French, with a London postmark, and Smith's full name anagrams to "U.R. Blanche Descartes, Limit'd". This quirky humour lasted throughout Smith's life, but never so light-heartedly as in his undergraduate years.
As a conscientious objector he spent the Second World War portering in a hospital. From1946 until his retirement he worked at the Galton Laboratory, University of London, where he became Weldon Professor of Biometry. His early work with J.B.S. Haldane applied the likelihood approach to human linkage, which he extended in highly original papers for the next half-century. Among Smith's achievements is the most powerful test for mimic loci, which produce what appears to be the same disease but are located in different chromosome regions and act in different ways. With James Renwick he pioneered sex-specific analysis based on the observation that chromosomes recombine at different points in male and female meiosis. Smith's 1953 paper introduced autozygosity mapping based on co-inheritance of a rare disease gene and close markers in relatives. The method lay fallow for thirty years, waiting for the molecular markers that have made it invaluable.
Smith's work was especially influential in the United States, which at the time he joined the Galton Laboratory had begun to develop the computers of which Charles Babbage had dreamed a century earlier. This stimulated applications that were unthinkable to Smith's predecessors in Britain, who dominated statistical genetics in the pre-computer era. Smith generously attributed the successes of the early computer period to his American competitors, who less characteristically gave him precedence. The truth is that the two currents were so intermixed and the rivalry so friendly as to baffle a historian of science. Smith's best ideas were incorporated into genetic mapping whereby hundreds of disease genes were localised as a necessary first step to sequencing, characterisation, and attempts to ameliorate their effects. This has been a precious tool for clinical genetics and the impetus for the Human Genome Project.
Although these contributions are best known, they are only a part of Smith's scholarship. He contributed to many of the classical topics in statistical genetics, including segregation ratios in family data, kinship, population structure, assortative mating, genetic correlation, and estimation of gene frequencies. The latter had wide application, but Smith's role has not been recognised by mathematicians. With Ceppellini and Siniscalco he introduced the method of gene counting in 1955. It gives maximum likelihood estimates that converge more slowly but more reliably than methods requiring an information matrix. This principle was presented at the Royal Statistical Society in 1976 by Dempster and others as the EM algorithm. Characteristically Smith contributed to the discussion without mentioning that he had priority of more than 20 years. The method is now used to advantage for many missing-data problems in which some of the observations are mixtures of discrete probabilities.
Smith's eminence in mathematics was recognised by membership in the Royal Statistical Society and the ISl. A large book has been written on "Squared Squares", the name under which the new branch of combinatorics founded by Smith and his small coterie is known in pure mathematics. Human geneticists, and especially genetic epidemiologists, appreciated his contributions to genetic statistics made when that field was nobbled by government policy that protected British computers. For a score of years the sciences that needed competitive computing were stifled, and many of their practitioners changed disciplines or countries. In that difficult period Smith continued his research, oblivious to practical problems. Fortunately his work was valued and the international effort benefited.
Until his wife's death Smith delighted his friends with a Christmas letter commenting on peculiarities of news, railway stations, and other English curiosities. He is survived by his son and remembered with affection by colleagues who overlapped his tenure of the Weldon Professorship and active retirement."[a]
Arthur Harold Stone (AHS) (1916-2000)

Arthur Stone
Arthur, the son of Jewish immigrant parents was born in Islington, near the Angel, in north London, England on September 30, 1916, and was educated at Christ's Hospital (a school in Sussex for children some of whom had lost one or both parents). When CABS first met AHS, they lived only five minutes walk from each other. AHS was living with his sister and mother. He earned a mathematical scholarship to Trinity. He graduated first class and was awarded a B.A. in 1938. He was awarded a Research Scholarship and received a M.A. degree in 1939. That same year AHS received a Visiting Fellowship at Princeton, so he arrived there as war broke out. From 1939 to 1941, he pursued doctoral studies, earning a Ph.D. in general topology under the supervision of Dr. Lefschetz in 1941. While at Princeton, AHS invented the Flexagon and developed it further in collaboration with Feynman, Tuckerman and Tukey. Flexagons have been the subject of an article by Martin Gardner. His doctoral dissertation earned him a fellowship at trinity. From 1941-42 he spent a year at the institute for Advanced Study at Princeton. While at Princeton, he met and marrried an analyst who shared his interest in topology, Dorothy Maharam.
AHS then went to Purdue University from 1942-44. This was followed by "war work" at the Geophysical Laboratory of the Carnegie Institute in Washington, D. C. from 1944-45. Having earned a Fellowship at Trinity, he returned there from 1946-48. From Trinity, he went to Manchester University to assume a Lectureship from 1948-57. He was promoted to Senior Lecturer in 1957 and remained there until 1961. From 1961 to 1987 he was Professor of Mathematics at the University of Rochester, Rochester, New York and became emeritus on retirement in 1987. Since 1988, AHS has been Adjunct Professor at Northeastern University in Boston, Massachusetts. During his notable career, AHS has held brief visiting positions at Colombia in 1961, Yale 1965-66 and Australian National University at Canberra in 1978. The Stones had two children, David and Ellen, who became mathematicians as well.
Obituary. Arthur Harold STONE (1916–2000),by P. Cohn
Arthur Harold Stone, who died on 6 August 2000, was one of the foremost general topologists of his time, and made significant contributions to a number of different parts of general topology. He had been a member of the [London Mathematical] Society since 1948. His parents were Simon and Rosa Petrescu who came from Galatz (later Galati), Romania, where his father was a civil engineer, working in Bulgaria. They had two daughters, but when the father lost his job in Bulgaria as a result of the Balkan war, of 1912-13, the family decided to emigrate to England, where they anglicized their name to Stone. Their son, Arthur Harold Stone, was born in London on 30 September, 1916. He grew up in Sherriff Road and attended the local school, but in 1927 won an LCC Scholarship to Christ's Hospital (Horsham). This was a boarding school which had had such successful pupils as Philip Hall, Christopher Zeeman (later Sir Christopher), and D.G. Northcott (Stone's contemporary). The mathematics teaching was in the hands of C.A.J. Trimble, himself a Wrangler. Here, Arthur won prizes in almost all subjects except sports (though he was also good at rugger).
In 1935 he gained a major scholarship to Trinity College, Cambridge. He excelled at the academic subjects, but was also an outstanding violinist and good at chess. At Cambridge he continued with the violin and became leader of the orchestra of the Cambridge Music Society. He was a Wrangler, and took his BA in 1938, before going to Princeton, to work for a PhD under S. Lefschetz. Although a single-minded mathematician, he had wide-ranging interests, and this combination often showed up in unexpected ways. To fit the American notebook sheets into his English binder, he had to trim off an inch of paper, and he began to fold these strips in various ways. This led to some interesting figures, which later became famous as 'flexagons' (see 6, 3). He was both very inventive and also adept with his hands, talents which he used in building a counterclockwise grandfather clock.
Another problem that occupied him and some of his friends, was how to dissect a square into unequal smaller squares. They managed a dissection with 69 squares, and this led to his first (joint) paper [1]. The method was criticized by Bouwkamp <1> , but this was later retracted, and in [5] the authors deal with Bouwkamp's criticism, and give a formula for their example in Bouwkamp's notation.
Later, Stone returned to graphs in [35], where he showed how Lichtenbaum's conjecture on the density of an n-dimensional normal space can be reduced to a question in graph theory.
In [60], he and the authors of [1] look at electrical networks as graphs, and give a determinantal expression for the current flow.
A similar problem, but with a more topological flavour, was generalizing the 'sandwich' theorem. Ulam had shown how to bisect three sets in space, of finite outer measure, by a plane. In [2], Stone and Tukey generalized the problem to n subsets of any set R with Caratheodory outer measure, where the plane is now replaced by an appropriate real function.
Stone's main interest was point-set topology, where he wrote on metrizable and paracompact spaces, unicoherent and multicoherent spaces, and modifications of compact spaces, Borel sets, rectangular tilings and various kinds of continuous functions. A paper with a great and lasting influence is [7], where he solves a problem raised by Dieudonne, by proving in a most elegant way that all metrizable spaces are paracompact -- by showing that paracompactness is equivalent to full normality, which was known to follow easily from metrizability. This proof was far from trivial, and the methods have played a significant role in later work done by others.
Another interesting result proved here is that the product of uncountably many copies of the integers is not normal, which (combined with the previous theorem) shows that a product of metrizable spaces is normal (or paracompact) if and only if at most countably many factors are non-compact. This led him to a study of unicoherent spaces; in [8] he established various conditions for unicoherence and proved relatins between subsets, their frontiers and intersections, often best possible. He continued this work in [9] and [10], where he showed that some expected generalizations break down in the multicoherent case, and found valid (but more complex) generalizations, and extensions of the Phragmen-Brouwer theorem [11], and studied an infinite degree of multicoherence.
In 1948 he made a brief excursion into fluid dynamics in [6], when he discussed the theoretical basis, validity and uniqueness of calculations by Kopel of flow past yawing cones, and treated second-order effects in [12].
Another excursion, into abstract sets, resulted in a paper with G. Higman [15], where they constructed an inverse system of non-empty sets with empty inverse limit; it had been known that for compact sets this limit must be non-empty (see <2> ). Stone returned to the topic in [45], where he gave conditions for the inverse limit of compact non-Hausdorff spaces to be non-empty, compact or hereditarily compact. Another paper along these lines is [30], wher he gave a criterion for a preordered set to have a partition into k cofinal subsets: each element must have at least k successors. The proof is an elaborate use of transfinite induction.
In 1942, in Pittsburgh, he married Dorothy Maharam, who was also a mathematician, working in measure theory, where she obtained some notable results and also did some joint work with her husband, resulting in a fruitful blend of general topological methods applied to measure-theoretic questions [43,44,46,47,52]. After the war he returned with his wife to England, where he was fellow of Trinity College, Cambridge from 1946 to 1948, and then went as lecturer to Manchester University.
He was a superb expositor, both in his papers and in his lectures, which were distinguished by their clarity. In 1952 his wife also joined the staff of the University.
In 1953 he made a study of the Boltyanskii density of a space. This was known to be at least 6 for any two-dimensional compactum. Stone proved that for any two-dimensional normal space it did not exceed 7, and gave examples where the value 6 was realized, using the nerve of the covering. He next returned to metrizability problems. Yu Smirnov had proved in 1956 that a locally countably compact Hausdorff space which is the union of ?.? many separable metric spaces is itself metrizable. Stone in [17] obtained analogous results without assuming the subsets to be separable. This led him in [18] to a study of the number of closed subsets of a metric space of a given cardinality and weight (equal to the least cardinal of a basis). These results were remarkable because in the Cech compactification of Z, a closed set is either finite or of cardinal 2. He went on, in [19], to discuss the existence of universal spaces in the class of all metric uniform spaces of density character m with conditions placed on the uniformity. In [21], he studied topological spaces in which each subspace is compact. The interesting examples of such spaces are not T. 2; they have been studied in connexion with algebraic constructions.
In 1961, the Stones went back to the USA, where they both obtained professorships at the University of Rochester, NY. Stone now turned to the study of Borel sets and analytic sets in metric spaces. He showed in [22] that a metric space is an absolute F. sigma if and only if it is locally compact. Since 'locally compact' implies 'sigma-compact', this includes the classical characterization of F. sigma-spaces by sigma-compactness. A detailed study of Borel sets appeared in [23], where he attacked the question of whether Borel isomorphisms are equivalent to generalized homeomorphisms, by an examination of cases where this is so. He continued his efforts to classify topological properties of Borel spaces preserved by Borel isomorphisms in [27]. For sigma-discrete spaces this leads to a solution of the classification problem. Later, in [39], he treats the problem of finding properties of absolute analytic spaces invariant under Borel isomorphisms, using the weight of the space. The current progress on absolute Borel sets and k-analytic sets in metric space is summarized in [34]. There follows a series of papers in which various properties of subsets of topological spaces, such as compactness, measurability, and so forth, are characterized.
In a paper with E. Michael [32], which has been described as 'very interesting and significant', he studies continuous images of the space of irrationals. they show that a metrizable space which is a continuous image of the space P of irrational numbers is also a quotient of P; in particular, the space of rationals is a quotient of the space of irrationals.
In 1982, the University of Rochester held a conference in Stone's honour <5>. Five years later he and his wife retired and went to Northeastern University, Boston, MA, where Arthur became Adjunct Professor in 1988. This was a part-time appointment, and it allowed them to spend each winter in England. He had been in good health (in fact, the couple regularly used to walk the three miles to the University and back home) until 2000, when in March he had an operation to remove an aneurism; he recovered well, but on August 6 died from ideopathic pulmonary fibrosis. The Stones had a son and a daughter, who both became mathematicians. I am greatly indebted to his widow and his daughter for providing information about the family. A number of mathematicians and former colleagues have also helped me in writing this obituary: P.J. Hilton, E.A. Michael, D.G. Northcott, S. Rosenbaum, C.A.B. Smith, F. Smithies, W.T. Tutte and J.E.G. Utting. I should like to express my warmest thanks to them here.
University College London. P.M. Cohn.
William Thomas Tutte (WTT) (1917 - 2002)

W.T.Tutte
The following biographical sketch is by Dan Younger (2002) [12];
"Bill Tutte was born May 14, 1917 at Fitzroy House in Newmarket, England. His father was the House gardener, his mother the caretaker. How he rose from this background to graduate from Trinity College, Cambridge, is as remarkable a chapter of his life as those that followed.
Fitzroy House is now, and was perhaps then, a horseracing stable. In Bill's first years, the family moved about with the vagaries of domestic employment until, when he was about three, they came to live in a house called Moor End, located high on the Yorkshire moor, in Aislaby, overlooking the Esk river, about three miles from Whitby and the east coast of England. His parents were the caretakers at Moor End. This is the place of first remembrances, where he first went to school. Then, only a few months after his beginning school, the family moved once again, back to the Newmarket area, to a little village Cheveley, three miles east of Newmarket centre. It so happens that the city of Cambridge lies fifteen miles to the west of Newmarket.
The family lived in a flint cottage, one half of a duplex, reached by a footpath adjacent to the 600 year old Anglican Church that dominates Cheveley. His father soon obtained the position of gardener at the Rutland Arms Hotel in Newmarket. This finally gave stability to family life: they lived in that cottage until his father's death in 1944.
Bill went to the village school, run by the Anglicans, from age six until eleven. He has spoken of the school as enlightened in its religious education and the possessor of a fine children's encyclopedia, which he frequently consulted. Aided by its contents, Bill developed a keen interest in astronomy.
He was a successful student: at age ten he took the scholarship examinations for secondary school. The schools in England were organized on a county basis and, through an ancient oddity of district boundaries, Cheveley lies in Cambridgeshire, even though Newmarket, in Suffolk, lies between Cheveley and the city of Cambridge. So when Tutte won a scholarship, it was to the distant school in Cambridge. Too distant, his parents judged, and he was kept at home. A year later he again took the examinations, with the same success. This time, at eleven, his parents took the headmaster's advice to enroll him at the Cambridge and County High School for Boys. It was to be for Bill a long daily commute, taking his bike into Newmarket and, if the weather was fair, on the further fifteen miles into Cambridge. Or, if it was foul, then take the train from Newmarket, with another mile's walk from the station at the far end.
In this school he further excelled in studies. A testament to this is a little shelf of books left in his office, each imprinted in gold on the cover with the school motto "Virtute et Fide" and with a plate inside inscribed by the headmaster. "Prize for Mathematics, Form VI", reads the inscription of "Shakespeare's Complete Works"; for "The Plays of John Galsworthy", it is "Prize for Chemistry, Form VI."
In 1935, he entered Trinity College, Cambridge. For his studies there he was, in his words, "adequately supported financially by a State Scholarship, a College Scholarship, and a grant from the County." As an undergraduate, Bill majored in chemistry. Indeed, he achieved First Class Honours therein, and went on to graduate study in that field. His first two publications describe experimental work in chemistry. But the evidence is clear that his primary interest, dating at least from his high school days, was in mathematics, though not perhaps in the fields most in vogue. From his first days at Cambridge, he participated regularly in the meetings of the Trinity Mathematical Society. There he met Cedric Smith, and then Leonard Brooks and Arthur Stone, like-minded undergraduates with whom he conducted researches in mathematics. They were mathematics majors; Bill formed a bond with these three that would remain close throughout their lives.
Not the first that they studied, but the one most remembered by history, was the problem of determining whether or not a square can be partitioned into smaller squares, all unequal in size. It arises from puzzle #40, Lady Isabel's Casket, in H.E. Dewdney's book "The Canterbury Puzzles".
It is, of course, not the puzzle but what they made of it that is remarkable. To begin they translated the problem to the language of electrical networks. In the 1940 paper that later described their work are formulas for electrical network functions, not just those found earlier by Kirchoff, but new ones for transfer functions. This paper became a standard reference for electrical network practitioners. The question of squaring the square, in electrical terms, became a study of rotational symmetry of a part of the network, and how reflection of the symmetric part can alter its currents without affecting potentials on its boundary. The level at which they conceived the problem is remarkably deep. Their method did succeed in finding partitions of squares into smaller unequal squares.
This was not the only memorable study that Tutte undertook in his initial period at Trinity, but the others were published later. For Tutte's academic career was put on hold by World War II.
In January 1941, upon invitation of his Tutor, Tutte went to Bletchley Park, the now legendary organization of code-breakers of Britain. It was later that year, in October, that Tutte encountered TUNNY, the first of a set of machine-ciphers named Fish. Now Fish is not Enigma. The Bletchley code-breakers, among whom Alan Turing was prominent, had had success in deciphering Enigma codes. But that success was with the naval and air force versions; the army version of Enigma proved to be resistant to analysis. That Bletchley could not read Army Enigma gave them incentive to attack Fish, which was used only by the Army. Moreover, Fish was used for high level communications between Berlin and the field commanders.
Tutte's great contribution was to uncover, from samples of the messages alone, the structure of the machines which generated these codes. This came about as follows. In August 1941, a German operator sent a Fish-enciphered teleprinter message of some 4000 letters from Athens to Berlin. For some reason, the message was not received properly and so it was resent. Against all guidelines, it was sent with the same setting. It was identical in content, but it differed slightly, in word spacing and punctuation. John Tiltman of Bletchley was able to use this blunder to find both the message and the obscuring string that was added to make up the enciphered message. But that seemed to be all that could be found, when Tutte was presented with the case in October.
Tutte began by observing the machine generated obscuring string carefully. Splitting it up into various lengths, he noticed signs of periodicity. For the first of the five teleprinter tape positions, the regularity he supposed arose from a wheel of 41 sprockets. And then at the last position, one of 23 sprockets. Over the next months, Tutte and colleagues worked out the complete internal structure, that it had twelve wheels, two for each of the five teleprinter positions, and two with an executive function. They determined the number of sprockets on each wheel, and how the advancement of the wheels was interrelated. They had completely recreated the machine without ever having seen one. Tony Sale, who first described this work in a 1997 article in New Scientist, characterized it as the "greatest intellectual feat of the whole war."
Knowing the structure of the enciphering machine is a necessity for code-breaking, but it is only the first step. Tutte then put himself to creating an algorithm to find from the enciphered messages the initial settings of the machine wheels. The algorithm that he created, the "Statistical Method", looked for certain types of resonances, but it had to consider far too many possibilities to be carried out by hand. So it was that, in 1943, the electronic computer COLOSSUS was designed and built by the British Post Office. It was to run the algorithms that Tutte; and his collaborators Max Newman and Ralph Tester; developed, that COLOSSUS was created. This man-machine combination was used to break Fish codes on a regular basis throughout the remainder of the War. Colossus was the first of the electronic digital machines to feature limited programmability.[9]
In late 1945, Tutte resumed his studies at Cambridge, now as a graduate student in mathematics. He published some work begun earlier, one a now famous paper that characterizes which graphs have a perfect matching, and another that constructs a non-Hamiltonian graph. He went on to create a ground-breaking Ph.D. thesis, "An algebraic theory of graphs", in which he forges the subject now known as matroid theory.
Upon completing his degree, Tutte was invited by H.S.M. Coxeter to come to Canada, to join the Faculty of the University of Toronto. In his fourteen years at Toronto, beginning in 1948, he rose to preeminence in the field of combinatorics. One form of recognition in that period was his election as Fellow of the Royal Society of Canada.
It is difficult to describe in a summary way the many branches of research that Tutte pursued. Fortunately, Tutte has himself given such a summary, in his comments in the "Selected Papers of W.T. Tutte", published in 1979, and "Graph Theory as I have known it", in 1998. It is notable the degree to which these researches have their point of origin in squaring the square and matroids.
In 1962, Tutte joined the Faculty of the University of Waterloo. This was just five years after its creation. Tutte made a major contribution to establishing the identity and reputation of the University. His presence was a magnet for combinatorialists from all over the world. It was not only recognized stars of the field that came to Waterloo, but those of future prominence. Tutte was an important ingredient in the recipe that produced the Faculty of Mathematics in 1967, becoming one of the first members of the Department of Combinatorics and Optimization. He retired in 1985, but continued to be a significant member of the Faculty as Professor Emeritus. Until his retirement, he was Editor in Chief of the Journal of Combinatorial Theory.
Bill Tutte enjoyed hiking, and soon after his arrival in Canada became a member of the Canadian Youth Hostels Association. It was through the Hostel movement that he met Dorothea Mitchell, from Oakville: they were married in October 1949. Dorothea was lively and chatty, Bill more reserved in manner: they formed a lovely couple. When they moved to Waterloo, they lived out in the little village of West Montrose, just adjacent to the wooden covered bridge that is the signature of the region. Here the Tuttes managed an extensive garden, of mostly wild flora, on the banks of the Grand River. The neighbourhood is a pleasant place for hikes. Dorothea was an avid and skilled potter, well-known for her pivotal role in the founding of the Waterloo Potters workshop. For the Waterloo Combinatorics Conference of 1968, she made a personalized mug for each of the invited speakers, some thirty five in all. Dorothea died of cancer in 1994; they had no children.
Here is an assessment of Tutte's impact by two leading combinatorialists.
Paul Seymour of Princeton University writes:
"Professor Tutte has been for many years the dominant figure in graph theory, and his contributions to the subject outweigh those of any other individual (in every sense except perhaps quantity). There are numerous instances when Tutte has found a beautiful result in a hitherto unexplored branch of graph theory, and in several cases this has been a ´breakthrough´, leading to the development of a major new subject."
Lászlo Lovász of Microsoft writes:
"Few theorems in mathematics are honored by the general public by naming them after the mathematician who proved them. In Tutte's case, however, there are several such results: for somebody working in matching theory, Tutte's theorem is his characterization of graphs having a perfect matching for a matroid theorist, it means his characterization of regular matroids for somebody studying Hamiltonian cycles it means his result that 4-connected planar graphs have a Hamilton cycle. And there is also the Tutte polynomial of a graph (and a matroid), which is again a household word for many combinatorialists."
Tutte was a master of phrasing: here are some examples. To begin, there is the phrase "squaring the square". Next, the term "wheel" is an apt description of the structure that lies at the heart of his analysis of 3-connected graphs; its nongraphical analog in matroid theory is "whirl". The title of a seminar describing a fatal flaw he had found in a famous mathematician's paper on 3-colouring was "Et tu, Tut-té!" The title of his famous paper on the Reconstruction Conjecture for graphs is "All the king's horses". In his penetrating analysis and reformulation of the Birkhoff-Lewis equations, he declares some of those equations to be "of mysterious provenance." Finally, one of his last public lectures was "Sixty years in the nets."
Tutte was awarded the Tory Medal by the Royal Society of Canada in 1975. He won the Killam Prize in 1982. Last year he was awarded the CRC-Fields Institute Prize; receiving this became the occasion for two of his last public lectures, the one referred to above in Toronto, the other in Montreal.
William T. Tutte, Distinguished Professor Emeritus at the University of Waterloo, died May 2, 2002. The cause was congestive heart failure, complicated by lymphoma of the spleen, both diagnosed within six weeks of his death.
It was just the year before, October 2001, that he was inducted as an Officer in the Order of Canada, in a ceremony held at Rideau Hall in Ottawa. The citation began "He is internationally renowned for his seminal work in the area of graph theory. As a young mathematician and codebreaker, he deciphered a series of German military encryption codes known as Fish". These two sentences speak to his place in history. His wartime codebreaking work, much emphasized in newspaper obituaries, was a secret by order of British security until 1993 and, indeed, is only described with detail in recent articles.
In 1987 Tutte was named a Fellow of the Royal Society of London."; Dan Younger, August 2002.
Brooks, Smith, Stone & Tutte (Part II)
In part II, William Tutte retells the story of how the square was squared.
References R.L. Brooks
- 'Squared Squares, Who's Who & What's What', Jasper Dale Skinner (1993) 14-49
- Mathworld - Brooks' Theorem
Works of R.L. Brooks
- (with C.A.B. Smith, A.H. Stone and W.T. Tutte) 'The Dissection of Rectangles into Squares', Duke Math. J. 7 (1940) 312–340.
- 'On Coloring the Nodes of a Network.' Proc. Cambridge Philos. Soc. 37, (1941) 194-197.
- 'A procedure for dissecting a rectangle into squares, and an example for the rectangle whose sides are in the ratio 2:1', J. Combinatorial Theory, 10, (1971) 206-211
- 'Determinants and Current Flows In Electric Networks', R. L. Brooks, C. A. B. Smith, A. H. Stone and W. T. Tutte, Discrete Mathematics 100, (1992) 291-301 * This paper is intended to celebrate not only the 100th anniversary of Petersen's paper, but also (approximately) the 50th anniversary of the original paper by Brooks, Smith, Stone and Tutte.
References C.A.B. Smith
- Cedric Smith (1917-2002) - Obituary by Newton Morton.
- Blanches Dissection - Mathworld entry
- 'Cedric Smith - a mathematical castaway'
by Tony Crilly, (2003) The Mathematical Gazette (UK) 18, - 'View from the lab: a mastermind for a number of reasons'
- Obituary by Steve Jones, (2002) UK Telegraph
Works of C.A.B. Smith
- (with R.L. Brooks, A.H. Stone and W.T. Tutte) 'The dissection of rectangles into squares'. Duke Math. J. 7 (1940) 312–340.
- 'Authors' EUREKA 45 (1985) 48
- C. A. B. Smith, Did Erdös save Western Civilization? , in: The Mathematics of Paul Erdös I, Algorithms and Combinatorics 13, pp. 74-85, Springer, 1997.
- (with J.D. Skinner and W.T. Tutte) 'On the Dissection of Rectangles into Right-Angled Isosceles Triangles.' J Comb. Theory Ser. B 80 2 (2000) 277-319
References A.H. Stone
- C.J. Bouwkamp, 'On the construction of simple perfect squared squares', Nederl. Akad. Wetensch. Proc. 50 (1947) 72-78; Indag. Math. 9 (1947) 57-63.
- S. Eilenberg and N. Steenrod, 'Foundations of algebraic topology', Princeton Math. Ser. 15 (Princeton University Press, 1952).
- M. Gardner, 'Hexaflexagons and other mathematical diversions' (University of Chicago Press, 1959/1988).
- I. Gessel, 'Trees and power sums', Letter to the Editor, Amer. Math. Monthly 93 (1986) 323-324.
- J.R. Harper and R. Mandlebaum, eds, 'Combinatorial methods in topology and algebraic geometry. Proceedings of a conference in honour of Arthur H. Stone', University of Rochester, Rochester, NY, June 29-July 2, 1982, Contemp. Math. 44 (Amer. Math. Soc., Providence, RI, 1985).
- 'Paper folding', Encyclopaedia Britannica, 15th edn (H.H. Benton, Chicago, 1974).
- W.W. Comfort interviews Arthur Stone (1996)
- Obituary, Arthur Stone (1916-2000), The London Mathematical Society 2002
Works of A.H. Stone.
- (with R.L. Brooks, C.A.B. Smith and W.T. Tutte) 'The dissection of rectangles into squares'. Duke Math. J. 7 (1940) 312–340.
- (with J.W. Tukey) 'Generalized "sandwich" theorems', Duke Math. J. 9 (1942) 356-359.
- (with P. Erdős) 'Some remarks on almost periodic transformations', Bull. Amer. Math. Soc. 51 (1945) 126-130.
- (with P. Erdős) 'On the structure of linear graphs', Bull. Amer. Math. Soc. 52 (1946) 1087-1091.
- (with R.L. Brooks, C.A.B. Smith and W.T. Tutte) 'A simple perfect squared square', Neder. Akad. van Wetensch. Proc. 50 (1947) 1300-1301; Indag. Math. 9 (1947) 626-627.
- 'On supersonic flow past a slightly yawing cone', J. Math. Phys. 27 (1948) 67-81. Corrections ibid. 31 (1953) 300.
- 'Paracompactness and product spaces', Bull. Amer. Math. Soc. 54 (1948) 977-982.
- 'Incidence relations in unicoherent spaces', Trans. Amer. Math. Soc. 65 (1949) 427-447.
- 'Incidence relations in multicoherent spaces I', Trans. Amer. Math. Soc. 66 (1949) 389-406.
- 'Incidence relations in multicoherent spaces II', Can. J. Math. 2 (1950) 461-480.
- 'Incidence relations in multicoherent spaces III', Pacific J. Math. 2 (1950) 99-126.
- 'On supersonic flow past a slightly yawing cone II', J. Math. Phys. 30 (1952) 200-213.
- 'On infinitely multicoherent spaces', Quart. J. Math. Oxford. Ser. (2) 3 (1952) 298-306.
- 'On coverings of two-dimensional spaces', Proc. London Math. Soc. (3) 3 (1953) 338-349.
- (with G.Higman) 'On inverse systems with trivial limits', J. London Math. Soc. 29 (1954) 233-236.
- 'Metrizability of decomposition spaces', Proc. Amer. Math. Soc. 7 (1956) 690-700.
- 'Metrizability of unions of spaces', Proc. Amer. Math. Soc. 10 (1959) 361-366.
- 'Cardinals of closed sets', Mathematika 9 (1959) 99-107.
- 'Universal spaces for some metrizable uniformities', Quart. J. Math. Oxford Ser. (2) 11 (1960) 105-115.
- 'Sequences of coverings', Pacific J. Math. 10 (1960) 689-691.
- 'Hereditarily compact spaces', Amer. J. Math. 82 (1960) 900-916. Errata, ibid. 86 (1964) 888.
- 'Absolute F. sigma-spaces', Proc. Amer. Math. Soc. 13 (1962) 495-499.
- 'Non-separable Borel sets', Rozprawy Mat. 28 (1962) 41 pp.
- 'Non-separable Borel sets', General topology and its relations to modern analysis and algebra, Proc. Sympos. Prague 1961 (Academic Press, New York/Publ. House Czech Acad. Sci., Prague, 1962) 341-342.
- 'A note on paracompactness and normality of mapping spaces', Proc. Amer. Math. Soc. 14 (1963) 81-83.
- 'Kernel constructions and Borel sets', Trans. Amer. Math. Soc. 107 (1963) 58-70.
- 'On sigma-discreteness and Borel isomorphism', Amer. J. Math. 85 (1963) 655-666.
- (with K.A. Ross) 'Products of separable spaces', Amer. Math. Monthly 71 (1964) 398-403.
- (with C.T. Scarborough) 'Products of nearly compact spaces', trans. Amer. Math. Soc. 124 (1966).
- 'On pertitioning ordered sets into cofinal subsets', Mathematika 15 (1968) 217-222.
- 'Disconnectible spaces', Proc. Topology Conference, Arizona State Univ. Tempe, AZ, 1967 (Arizona State Univ., Tempe, AZ, 1968) 265-276.
- (with E. Michael) 'Quotients of the spaces of irrationals', Pacific J. Math. 28 (1969) 629-633.
- (with P. Erdős) 'On the sums of two Borel sets', Proc. Amer. Math. Soc. 25 (1970) 304-306.
- 'Borel and analytic metric spaces', Proc. Washington State Univ. Conf. on General Topology, Pullman, WA, 1970 (Pi Mu Epsilon, Dept. of Math., Washington State Univ., Pullman, WA, 1970) 20-33.
- 'Some combinatorial problems in general topology', Combinatorial structures and their applications, Proc. Calgary Internat. Conf., Calgary, AB 1969 (Gordon and Breach, New York, 1970) 413-416.
- (with F.B. Jones) 'Countable locally connected Urysohn spaces', Colloq. Math. 22 (1971) 239-244.
- (with K.P. Rajappan) 'On Okada's method for realizing cut-set matrices', J. Combin. Theory, Ser. B. 10 (1971) 113-134.
- 'Unions of locally compact spaces', Proc. University of Houston Point Set Topology Conference, Houston, TX, 1971 (Univ. Houston, TX, 1971) 56-75.
- 'Non-separable Borel sets II', General Topology and Appl. 2 (1972) 249-270.
- 'Some problems of measurability', Proc. Topology Conference, Virginia Polytech. Inst. and State Univ., Blacksberg, VA, 1973, Lecture Notes in Math. 375 (Springer, Berlin 1974) 242-248.
- 'Topology and measure theory', Measure theory, Proc. Conf. Oberwolfach, 1975, Lecture Notes in Math. 541 (Springer, Berlin, 1976) 43-48.
- 'Measure-preserving maps', General topology and its relations to modern analysis and algebra IV, Proc. Fourth Prague Topological Sympos., Prague, 1976, Part A, Lecture Notes in Math. 609 (Springer, Berlin, 1977) 205-210.
- (with D. Maharan) 'Realizing isomorphisms of category algebras', Bull. Austral. Math. Soc. 19 (1978) 5-10.
- (with D. Maharam) 'Borel boxes', Pacific J. Math. 81 (1979) 471-473.
- 'Inverse limits of compact spaces', General Topology Appl. 10 (1979) 203-211. Erratum, ibid. 11 (1980) 335.
- (with D. Maharam) 'Category algebras of complete metric spaces', Mathematika 26 (1979) 13-17.
- (with D. Maharam) 'One-to-one functions and a problem on subfields', Measure theory, Oberwolfach 1979, Proc. Conf. Oberwolfach, 1979, Lecture Notes in Math. 794 (Springer, Berlin, 1980) 49-52.
- 'Absolutely FG spaces', Proc. Amer. Math. Soc. 80 (1980) 515-520.
- 'Analytic sets in non-separable metric spaces', Analytic sets, Proc. Conference at University College London, 1978 (Academic Press, London, 1980) 471-480.
- (with R. Daniel Mauldin) 'Realizations of maps', Michigan Math. J. 28 (1981) 369-374.
- (with R. Daniel Mauldin) 'Realizations of maps', Measure Theory Oberwolfach 1981, Proc. Conf. Oberwolfach, 1981, Lecture Notes in Math. 945 (Springer, Berlin/New York, 1982) 145-149.
- (with D. Maharam) ' Expression measurable functions by one-to-one ones', Adv. in Math. 46 (1982) 151-161.
- 'Compact and compact Hausdorff', Aspects of topology, London Math. Soc. Lecture Note Ser. 93 (ed. I.M. James and E.H. Kronheimer, Cambridge University Press, 1985) 315-324.
- 'Trees and power sums', Amer. Math. Monthly 92 (1985) 328-331.
- 'Closed tilings of Euclidean spaces', Proc. Conf. commemorating the first centennial of the Circolo Matematico di Palermo (Palermo 1984), Rend. Circ. Mat. Palermo (2) Suppl. 8 (1985) 321-324.
- 'Borel sets in analytic spaces', Atti Sem. Mat. Fis. Univ. Modena 35 (1987) 135-140.
- (with E.K. van Douwen) 'The topology of close approximations', Topology Appl. 35 (1990) 261-275.
- 'The topology of close approximations', General topology and applications, Middletown, CT, 1988, Lecture Notes in Pure and Appl. Math. 123 (M. Dekker, New York, 1990) 263-268.
- 'Finite unions of locally nice spaces', Topology Appl. 41 (1991) 57-64.
- (with R.L. Brooks, C.A.B. Smith and W.T. Tutte) 'Determinants and current flows in electric networks', Discrete Math. 100 (1992) Special volume to mark the centennial of Julius Petersen's "Die Theorie der regularen Graphen", Part I, 291-301.
- 'The measurability of nonsingular transformations', Measure theory (Oberwolfach, 1990), Rend. Circ. Mat. Palermo (2) Suppl. 28 (1992) 41-42.
- (with E.K. van Douwen) 'Are most measurable functions one-to-one?', Portugal. Math. 49 (1992) 429-446.
- 'Covering dimension from large sets', Papers on general topology and applications (Gorham, ME 1995), Ann. New York Acad. Sci. 806 (New York Acad. Sci., New York, 1996) 438-443.
- 'A.H. Lusin's theorem', Atti Sem. Mat. Fis. Univ. Modena 44 (1996) 351-357.
- 'Encounters with Paul Erdős', The mathematics of Paul Erdős, I, Algorithms Combin. 13 (Springer, Berlin, 1997) 68-73.
- 'Some topologists of the 1940s', Handbook of the history of general topology, vol. 1 (Kluwer Acad. Publ., Dordecht, 1997) 105-109.
- 'sigma-fields of bad Borel sets', Topology Appl. 82 (1998) Special volume in memory of Kiiti Morita, 421-426.
References W.T. Tutte
- Wikipedia on Colossus
- Dan Younger's biographical sketch of Bill Tutte's life and achievements.(reproduced above)
- William T. Tutte (1917 -2002) Arthur M. Hobbs And James G. Oxley,
- Colossus: The Secrets of Bletchley Park. (William Tutte's role)
Works of W.T. Tutte.
- (with R.L. Brooks, C.A.B. Smith and A.H. Stone). 'The dissection of rectangles into squares'. Duke Math. J. 7 (1940) 312–340.
- 'On Hamiltonian circuits'. J. London Math. Soc., 21 (1946) 98-101.
- 'A family of cubical graphs'. Proc. Cambridge Phil. Soc. 43 (1947) 26-40.
- 'A ring in graph theory'. Proc. Cambridge Phil. Soc. 43 (1947) 26-40.
- 'The factorization of linear graphs'.J. London Math. Soc.22 (1947) 107-111.
- 'The dissection of equilateral triangles into equilateral triangles'. Proc. Cambridge Phil. Soc. 44 (1948) 463-482.
- Thesis, Cambridge (1948).
- 'On the imbedding of linear graphs in surfaces'.Proc. London Math. Soc. (2), 51 (1949) 474-483.
- 'Squaring the square'. Can. J. Math.,2 (1950) 197-209.
- 'The factors of graphs'.Can. J. Math.,4 (1952) 314-328.
- 'A contribution to the theory of chromatic polynomials'.Can. J. Math., 6 (1954) 81-90.
- 'A short proof of the factor theorem for finite graphs'.Can. J. Math., 6 (1954) 347-352.
- 'A class of Abelian groups'.Can. J. Math., 8 (1956) 13-28.
- 'A theorem on planar graphs'. Trans. Amer. Math. Soc., 182 (1956) 99-116.
- 'A homotopy theorem for matroids', I.Trans. Amer. Math. Soc., 88 (1958) 144-160.
- 'A homotopy theorem for matroids', II.Trans. Amer. Math. Soc. 88 (1958) 161-174.
- 'Matroids and graphs'.Trans. Amer. Math. Soc., 90 (1959) 621-624.
- 'On the symmetry of cubic graphs'. Can. J. Math., 11 (1959) 527-552.
- 'A non-Hamiltonian graph'.Can. Math. Bull., 3 (1960) 1-5.
- 'A non-Hamiltonian planar graph'. Acta. Math. Acad. Sci. Hung., 11 (1960) 371-375.
- 'An algorithm for determining whether a given binary matroid is graphic'.Proc. Amer. Math. Soc., 11 (1960) 905-917.
- 'Squaring the square'. In 2nd Scientific American Book of Mathematical Puzzles and Diversions, by Martin Gardner. (1961) New York.
- 'Symmetrical graphs and coloring problems'.Scripta Math., 25 (1961) 305-316.
- 'A census of Hamiltonian polygons'. Can. J. Math., 14 (1962) 402-417.
- 'A census of planar triangulations'. Can. J. Math., 14 (1962) 21-38.
- 'A census of slicings'. Can. J. Math., 14 (1962) 708-722.
- 'A census of planar maps'. Can. J. Math., 15 (1963) 249-271.
- (with W.G. Brown). 'On the enumeration of rooted non-separable planar maps'. Can. J. Math., 16 (1964) 572-577.
- 'Lectures on matroids'.J. Res. Nat. Bur. Standards, Sect. B, 69B (1965) 1-47.
- 'On dichromatic polynomials'.J. Comb. Theory, 2 (1967) 301-320.
- 'On the enumeration of planar maps.Bull. Amer. Math. Soc., 74 (1968) 64-74.
- 'On the enumeration of four-coloured maps'.SIAM J. Appl. Math., 17 (1969) 454-460.
- 'On chromatic polynomials and the golden ratio'.J. Comb. Theory, 9 (1970) 289-296.
- 'More about chromatic polynomials and the golden ratio'. Combinatorial structures and their applications, (ed R.K. Guy et al.) (1970) 439-453.
- 'Chromatic sums for rooted planar triangulations: the cases Λ = 1 and Λ = 2.' Can. J. Math., 25 (1973) 426-447.
- 'Chromatic sums for rooted planar triangulations II: the case Λ = Τ + 1.' Can. J. Math., 25 (1973) 657-671.
- 'Chromatic sums for rooted planar triangulations III: the case Λ = 3.' Can. J. Math., 25 (1973) 780-790.
- 'Chromatic sums for rooted planar triangulations IV: the case Λ = ∞' Can. J. Math., 25 (1973) 929-940.
- 'Chromatic sums for rooted planar triangulations V: special equations.' Can. J. Math., 26 (1974) 893-907.
- 'Duality and Trinity.'Colloq. Math. Soc. J. Bolyai, 10 (1973) 1459-1472.
- 'Codichromatic graphs.'J. Comb. Theory B., 16 (1974) 168-174.
- 'Spanning subgraphs with specified valencies.'Discrete Mathematics, 9 (1974) 97-108.
- (with R.L. Brooks, C.A.B. Smith and A.H. Stone). 'Leaky Electricity and triangled triangles'. Phillips Res. Reports, 30 (1975) 205-219
- 'The rotor effect with generalized electrical flows.'Ars Combinatoria, 1 (1976) 3-31.
- 'Bridges and Hamiltonian circuits in planar graphs.' Aequationes Math., 17 (1978) 121-140.
- 'On a pair of functional equations of combinatorial interest.' Aequationes Math. 17 (1978) 121-140.
- 'All the king's horses. A guide to reconstruction.' In Graph Theory and related topics, Academic Press, New York. (1979) 15-33.
- 'Selected papers of W.T. Tutte.' D. McCarthy and R.G. Stanton, eds. The Charles Babbage Research Centre, St. Pierre, Manitoba, Canada (1979).
- '1-factors and polynomials'.Europ. J. Combinatorics, 1 (1980) 77-87.
- 'Dissections into equilateral triangles'. In The Mathematical Gardner, ed. D.A. Klarner. Wadsworth International, Belmont, California, (1981) 127-139.
- 'Chromatic solutions'. Can. J. Math., 34 (1982) 741-758.
- 'Chromatic solutions II'. Can. J. Math., 34 (1982) 952-960.
- 'The method of alternating paths'. Combinatorica., 2 (1982) 325-332
- 'Graph Theory.' Addison-Wesley, New York (1984).
- 'From topology to algebra.' J. Graph Theory 10 (1986) 331-337.
- 'On the Birkhoff-Lewis equations.' Discrete Math. 92 (1991) 417-425.
- 'The matrix of chromatic joins.' J. Combin. Theory Ser. B 57 (1993) 269-288.
- 'Chromatic sums revisited.' Aequationes Math. 50 (1995) 95-134.
- 'Dichromatic sums revisited.' J. Combin. Theory Ser. B 66 (1996) 161-167.
- 'Graph theory as I have known it.' Oxford University Press (1998) New York.
- 'Bicubic planar maps.' Ann. Inst. Fourier (Grenoble) 49 (1999) 1095-1102.
- 'Fish and I.' Coding Theory and Cryptography, Springer Berlin. (2000) 9–17.
- (with J.D. Skinner and C.A.B. Smith) 'On the Dissection of Rectangles into Right-Angled Isosceles Triangles.' J Comb. Theory Ser. B 80 2(2000) 277-319