Michio Abe, Japan
In 1930 Michio Abe, published a paper "Covering the square by squares without overlapping," in the Journal of Japan Mathematical Physics. This paper has been cited in a number of recent (2002-2005) Japanese and Chinese publications on floorplanning and packing in VLSI placement design. This shows the topic and in particular, Abe's work still generates interest and has important practical applications.
Three things have been said about Abe's work;
- Working in apparent isolation, he produced over 600 simple perfect rectangles
- It appears he was aware of the literature of his day, in particular Z Moron´
- He was far ahead of the Germans in researching the topic
Abe published a second paper in 1931. An English translation was published in 1932. This was his last known paper, in it he showed that an infinite series of compound perfect rectangles can be built up (from a simple perfect rectangle [191 x 195]) whose size-ratios approach the limit of one. Little else is known about him and his work. One can only speculate as to whether Abe was caught up in the Japanese military, died or simply lost interest in the problem.
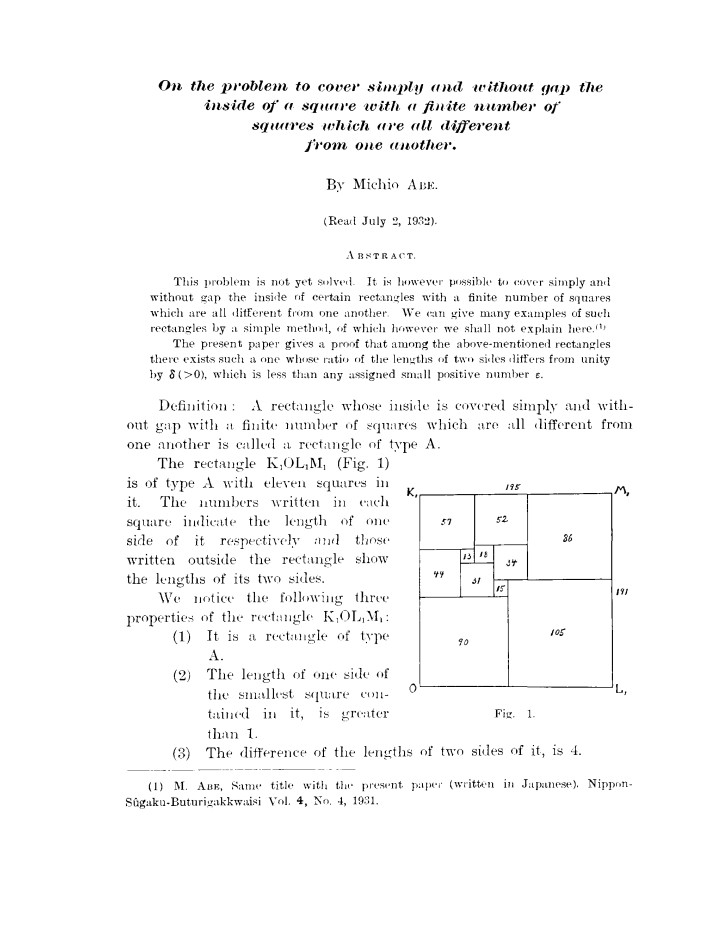
Michio Abe's 1932 paper
Sangaku
The Japanese have a rich culture of geometry and sangaku puzzle solving. In Kyoto there is a Sangaku of a rectangle dissected into 4 (non-trivial) right angle triangles. Perhaps Abe was inspired by a Sangaku, but no Sangaku of a squared square or squared rectangle has been found to my knowledge.
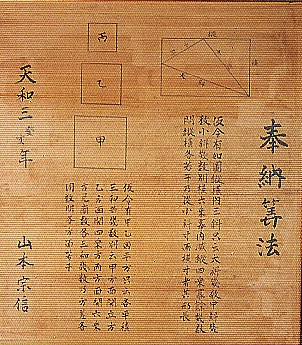
gokoumiya Sangaku (Kyoto Prefecture)
References
- "Covering the square by squares without overlapping," in the Journal of Japan Mathematical Physics, Vol.4, No.4, pp. 359--366, 1930 (in Japanese)
- "On the problem to cover simply and without gap the inside of a square with a finite number of squares which are all different from one another"., Proc Phys-Math. Soc. Japan 14 (1932) 385-387 (In English)
- 'Squared Squares, Who's Who & What's What', p13 Jasper Dale Skinner, II, Ph.D.