Sam Loyd

Sam Loyd
Always known as Sam, Samuel Loyd was the creator of famous mathematical puzzles and recreations. He was born in Philadelphia where his father was an estate agent, but he did not live there for very long since when he was only three years old his family moved to New York. Sam's father had a good income from being an estate agent and the family were well off. It was a large family with Sam being the youngest of nine children.
He attended school in New York, completing his secondary education in 1858. Already by this time he had become fascinated by the game of chess which he took up when he was 14 years old when he began to attend a chess club with his brothers Thomas and Isaac. While still at school, Loyd won prizes for his chess problems.
Loyd's first chess problem was published in the New York Saturday Courier on 14 April 1855. After leaving school Loyd began to study engineering, intending to become a steam and mechanical engineer, but he found he could make his living from his puzzles and chess problems.
Loyd's interests were by now rather diverse for as well as earning money as a plumbing contractor he had purchased a chain of music stores. In fact he had other skills such as that of wood engraver, which he taught himself, and he was also a skilled cartoonist. He continued to compose chess problems, write newspaper columns, and edit papers such as Sam Loyd's Puzzle Magazine and even a mechanics journal. He also played in chess tournaments although he was not a top class chess player.
He visited Europe in 1867 and played in a tournament in Paris but performed poorly, however while there he did meet many who were interested in composing chess problems. By 1870 Loyd had become more interested in composing mathematical puzzles than chess problems.
Loyd produced over 10,000 puzzles in his lifetime many involving sophisticated mathematical ideas.
Alain C White wrote Sam Loyd and his Chess Problems (1913) and in it he writes:-
Ideas came to [Loyd] with great fecundity, often too rapidly for him to analyse them completely. Yet his powers for rapid analysis were almost unrivalled. He could see an idea from many sides at once; first always from the point of view of a puzzle, then from the humorous standpoint, finally from the artistic aspect.
Mrs Perkins Quilt – The Patch Quilt Puzzle (1914 – 1917)
In 1914 The Patch Quilt Puzzle appeared in Sam Loyd: “Cyclopedia of Puzzles”, privately published by his son after his death (reprinted in “Mathematical Puzzles of Sam Loyd”, selected and edited by Martin Gardner, Vol 1, pp. 73, 147-148. Dover, New York, 1959).
A square quilt made of 169 square patches of the same size is to be divided into the smallest number of square pieces by cutting along lattice lines.
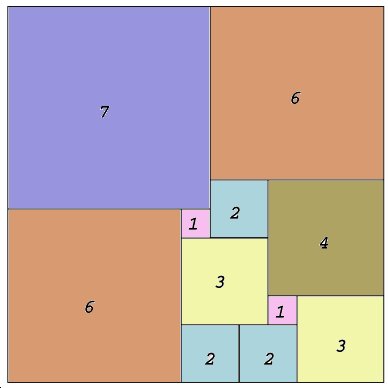
Mrs Perkins Quilt
The answer, which is unique, is composed of 11 squares with sides 1,1,2,2,2,3,3,4,6,6,7 . It is neither perfect nor simple.
In more recent terminology, Mrs Perkins Quilt's are known as imperfect squared squares (not all squares in the tiling are different sizes, hence the descriptor 'imperfect').
Gardner states that this problem first appeared in 1907 in a puzzle magazine edited by Sam Loyd. David Singmaster lists it as first appearing in 1914 in Cyclopedia by Loyd but credits Loyd with publishing Our Puzzle Magazine in 1907 - 08.[2]
This puzzle also appeared in a publication by Henry Dudeney as Mrs Perkins Quilt. Problem 173 in Amusements in Mathematics. 1917.
Mrs Perkins Quilt solution;
11 squares in a square of side 13. Sizes of squares 1, 1, 2, 2, 2, 3, 3, 4, 6, 6, 7
References
- MacTutor Biography for Sam Loyd
- Chronology Of Recreational Mathematics by David Singmaster